Mapping out the Fermi surface of KFe2As2

Angle-Dependent Magneto-Resistance (ADMR) is a powerful technique to map out the Fermi surface properties of a quasi-two-dimensional metal [see this earlier post]. I have worked on the theory of ADMR for more than a decade now and it continues to provide new challenges and applications to new classes of materials [see for example this talk].

I was delighted to see recently a PRL by an Aussie Rules football team [18 co-authors!] from Japan which has used ADMR to map out some of the Fermi surfaces for one of the new Iron Pnictide superconductors, KFe2As2 [these are in the Class II which exhibit some evidence for superconducting gap nodes].

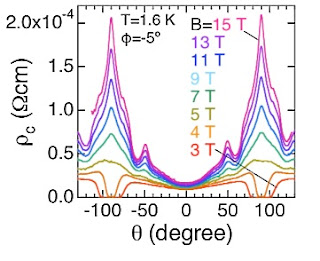
The figure below shows the angular dependence of the interlayer magnetoresistance at different magnetic field strengths.

This is the resulting two Fermi surfaces within the most conducting layers.

The authors note that the cross-sectional areas of the Fermi surfaces found by a range of techniques:
ADMR (12 and 17% of the FBZ),
one ARPES study (7 and 22%),
another ARPES (10, 12, and 29%),
dHvA (8 and 13%)
are all inconsistent with one another! 

The authors also state "no band structure calculation can reasonably reproduce the precise topology of the Fermi Surfaces of KFe2As2".

The authors assign the broad peak at theta = 90 degrees in the interlayer resistance as being evidence for coherent interlayer transport. I am not completely convinced of this. The strong angular dependence between 60 and 90 degrees is quite different from the weak angular dependence seen in traditional ADMR. For example, compare the figure above to the calculated ADMR shown below, taken from this PRB.