Wednesday, March 31, 2010
How to win a Nobel prize with a mean-field theory
A passion for science
I learned so many things from him just by observing how he ran the weekly brown bag lunches with his graduate students and later mine--listening with great interest as they described their research progress. He asked insightful and penetrating questions, but not in a threatening manner, made suggestions, and frequently went to visit the labs late in the day to see what was going on. He also hosted many undergraduate research students. He was devoted to education and student training and would prepare as carefully for lectures to freshman chemistry classes as for presentations to prestigious assemblages of scientists.
Tuesday, March 30, 2010
An equation you should know
Monday, March 29, 2010
Quantum dynamics of nuclear collisions
Sunday, March 28, 2010
Quantum chemistry par excellence
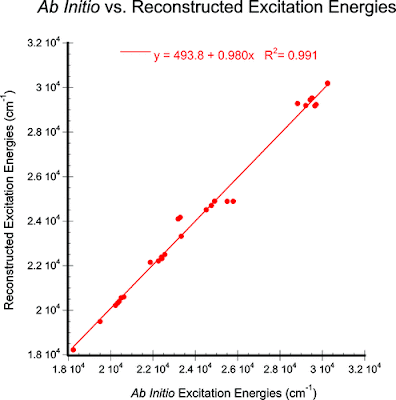
It is well known that ....
Friday, March 26, 2010
Deconstructing excitons in organic materials
Thursday, March 25, 2010
A great quantum many-body theorist
Wednesday, March 24, 2010
Problems with Hartree-Fock
Tuesday, March 23, 2010
Deconstructing Anderson's radical proposal?
"The preexisting magnetic singlet pairs of the insulating state become charged superconducting pairs when the insulator is doped sufficiently strongly."

Error bars please
- average scores on student evaluations of teaching
- impact factors of journals
- citation rates per paper

This site reports "large changes" in impact factors of Chemistry Journals from 2007 to 2008. It reports that of ChemPhysChem changed from 3.502 to 3.636.
Monday, March 22, 2010
Geometric frustration of kinetic energy


Sunday, March 21, 2010
Listen to the questions
Friday, March 19, 2010
Emergence of a hierarchy
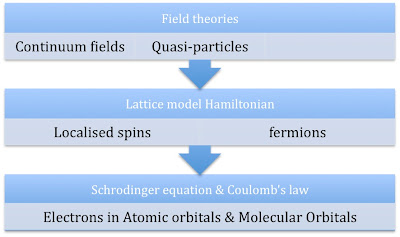
Wednesday, March 17, 2010
A good place to start a many-body theory
Tuesday, March 16, 2010
Want ad: a measure for quantum frustration


Monday, March 15, 2010
Trends in organometallic complexes


Sunday, March 14, 2010
How wrong about the future can you be? III
the era of the great breakthrough is over..... I have found that when I suggest to senior physicists that the end of physics as we know it is in sight, they tell me, "That's just what everybody was saying in 1900". Now this may be a justification for optimism, but let's first ask whether the historical parallel is sound. I think in many ways it is.
....If you don't believe me, ask yourselves this question: Apart from the field of fundamental particles, what is the most recent discovery in physics that still remains in essence a mystery? I think I might remark that in low-temperature physics the disappearance of liquid helium, superconductivity, and magneto-resistance from the list of major unsolved problems has left this branch of research looking pretty sick from the point of view of any young innocent who thinks he's going to break new ground.
....but with the new IBM Laboratory, and all those other labs that we represent, plugging along assiduously doing research, ten years is going to see the end of our games as pure physicists,....
Note, that the same time Pippard was giving this talk his very own Ph.D student, Brian Josephson was making a discovery that led to elucidation of spontaneously broken symmetry, macroscopic quantum tunneling, superconducting qubits, .....
Saturday, March 13, 2010
Galaxy formation as a condensation phenomena

Friday, March 12, 2010
One of my scientific heroes: John R. Platt (1918-1992)
Wednesday, March 10, 2010
To commute or not to commute
Tuesday, March 9, 2010
An alternative hypothesis for the cuprates
In Mike Norman's nice review of the theory of superconductivity in the cuprates he states:
The RVB spin gap was probably the first prediction for the subsequently observed pseudogap phase. In RVB theory, the pseudogap phase corresponds to a spin singlet state (with its resulting spin gap) but no phase coherence in the charge degrees of freedom. One of the interesting ideas to emerge from this was an explanation for transport in this phase, which reveals a metallic behavior for in-plane conduction, but an insulating behavior for conduction between the planes. In the RVB picture, the metallic behavior is due to the fact that the holons can freely propagate. But to tunnel between the planes, the holons and spinons must recombine to form physical electrons, and this costs the spin gap energy, thus one obtains insulating like behavior for the c-axis conduction (Lee, Nagaosa, Wen, 2006). This “gap” has now been directly seen in c-axis infrared conductivity data (Homes et al., 1993).
However, it seems to me that there is now an alternative hypothesis to explain the c-axis infrared conductivity data, which does not require this exotic recombination of holons and spinons into an electron. This is provided by a paper by Michel Ferrero, Olivier Parcollet, Gabriel Kotliar, and Antoine Georges, discussed in this earlier post. The difference between in plane and interplane transport arises simply from the wavevector dependence of the interplane hopping matrix element. But, the observed gap is still due to the pseudogap.
Excitation spectra of spin liquids and superconductors
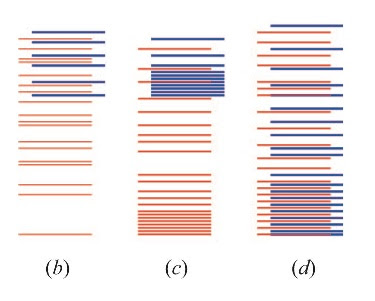
Monday, March 8, 2010
Sharing our ignorance...

Saturday, March 6, 2010
The future of quantum computing back then
Even if quantum computing remains a dream, the physics of quantum information processing at the level of a few qubits is fascinating. Experiments on entangled particles with ions in a trap or atoms in a cavity will help us understand the fundamental aspects of quantum measurement theory, and they may lead to major improvements in the precision spectroscopy of simple quantum systems..... Testing quantum decoherence in conceptually simple experiments is also an important and challenging task. Rather than teaching us how to build a large quantum computer, such experiments are more likely to teach us about the processes that would ultimately make the undertaking fail. It is important to advertise this fascinating subfield of quantum optics for what, it really promises, which is a deeper insight into the most counterintuitive theory yet discovered by physicists.
Tips on writing papers
- the emphasis that computational chemistry should be motivated by and ultimately connect with experiment
- the figures are key
Friday, March 5, 2010
Quantifying electronic correlations in simple metals
 For a non-interacting fermion system n(k)=1 for k less than 1 and 0 for k >1.
For a non-interacting fermion system n(k)=1 for k less than 1 and 0 for k >1.Thursday, March 4, 2010
How wrong about the future can you be?
Entanglement in quantum chemistry
Wednesday, March 3, 2010
Quick to save the planet

Simplifying the Theory of Everything
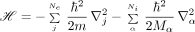

The symbols Zα and Mα are the atomic number and mass of the αth nucleus, Rα is the location of this nucleus, e and m are the electron charge and mass, r j is the location of the j th electron, and ℏ is Planck's constant.
Tuesday, March 2, 2010
Anderson's radical idea
Monday, March 1, 2010
A cautionary word about email
Thermodynamics and emergence
Novelty. Temperature and entropy are emergent properties. Classically, they are defined by the zeroth and second laws of thermodynamics, re...
-
Is it something to do with breakdown of the Born-Oppenheimer approximation? In molecular spectroscopy you occasionally hear this term thro...
-
I welcome discussion on this point. I don't think it is as sensitive or as important a topic as the author order on papers. With rega...
-
Nitrogen fluoride (NF) seems like a very simple molecule and you would think it would very well understood, particularly as it is small enou...


