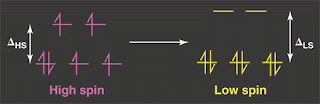In Telluride
Francesco Paesani gave a nice talk about his work on
metal-organic-frameworks, including those that exhibit
spin crossover.
The underlying physics is fascinating as there is a subtle interplay of electronic, magnetic, and lattice effects.
The crossover/transition is actually driven by changes in the vibrational entropy.
Spin "crossovers" involve a transition such as that shown above, from a S=2 state (HS=high spin) to a S=0 state (LS=low spin) often seen in Fe(2+) compounds.This transition occurs as the temperature is decreased, and sometimes is not a crossover, but a first order transition with hysteresis.
Spin crossover in metal-organic frameworks (MOFs) are of such great interest because the transition temperature can
- be comparable to room temperature
- vary significantly as the MOF (which is very porous) adsorbs "guest" molecules such as water.
These properties have significant technological potential but also involve some fascinating science I want to elucidate here.
First,
what determines the transition temperature?
In
a simple two state model it is given by the ratio of the energy (enthalpy) difference between the two states and the entropy difference between them,
The energy difference between LS and HS = 2U - 2J -2 Delta where
U = Hubbard interaction = Coulomb interaction in a single d orbital
J = Hund's rule interaction favouring parallel spin in degenerate orbitals
Delta = Crystal field splitting of d-orbitals (e.g. the t_2g - e_g splitting in an octahedral environment).
Typically, we would expect this energy difference to be a substantial fraction of an eV (i.e. thousand's of Kelvin), unless there is some amazing cancellation (fine tuning) going on. Indeed, as shown below our expectations our correct.
Also, given one is looking at the difference between large energies one should be cautious about getting accurate values of this quantity from quantum chemical methods.
A first guess for the entropy difference between the high and low spin states is the difference in spin entropy, k_B ln (5). This explains why the HS state occurs at high temperatures. However, taking this guess with that for the energy difference gives a transition temperature of thousands of Kelvin. So the entropy difference must be much larger and due to something else.
Theoretical Modeling of Spin Crossover in Metal–Organic Frameworks: [Fe(pz)2Pt(CN)4] as a Case Study
Jordi Cirera, Volodymyr Babin, and Francesco Paesani
The figure below shows the calculated energy of the HS and LS state versus delta = average value of the six metal-ligand bonds.
Three key observations are
- the energy gap between the two states is about 10 kcal/mol (0.4 eV corresponding to a temperature of about 5000 K).
- the low spin-high spin transition is associated with a significant lattice distortion of about 0.2 Angstroms.
- the high spin state is much "softer" (the parabola has a smaller curvature), i.e, the metal-ligand stretch frequency will be significantly smaller
The solid and dashed curves correspond to two different computational approaches. The latter is based on density functional theory (DFT). The former is empirical, ligand field force field (LF-FF), a method I previously
highlighted of value for modelling transition metal complexes in proteins.
I also note that the crystal field splitting (ligand field for chemists) Delta = 10,000 cm^-1 and
so 2 Delta = 2.5 eV and the HS-LS energy difference does involve subtle differences.
The harmonic vibrational frequencies for modes less than 500 cm-1 (comparable to room temperature) are calculated and it is found that these thirty modes are
softer in the HS state, leading to a higher entropy in the HS state. Using the associated entropy difference gives a value of the transition temperature that is comparable to experiment.
It is argued that these modes are softer because in the HS state the anti-bonding e_g orbitals are occupied and so the bonds are softer. I understand this argument for modes such as the metal-ligand modes. However, it is not clear to me why this matters for (the many) modes such as those largely localised on the ligands.
Thus, there is a theoretical understanding of the first outstanding property of spin crossover in MOFs: the relatively low transition temperature.
What about the second property: the dependence on adsorbed molecules?
Molecular Mechanisms of Spin Crossover in the {Fe(pz)[Pt(CN)4]} Metal–Organic Framework upon Water Adsorption
C. Huy Pham, Jordi Cirera, and Francesco Paesani
The decrease of the spin-crossover temperature of {Fe(pz)[Pt(CN)4]} upon water adsorption predicted by the simulations is in agreement with the available experimental data and is traced back to the elongation of the bonds between the Fe(II) atoms and the organic linkers induced by interactions of the adsorbed water molecules with the framework.
Again the key is the change in vibrational entropy. Upon adsorption the modes get softer.
There is more direct experimental evidence for
Role of Molecular Vibrations in the Spin Crossover Phenomenon
Jean-Pierre Tuchagues , Azzedine Bousseksou, Gábor Molnár, John J. McGarvey, François Varret
That review considers how IR, Raman, and specific heat measurements show softening of a wide range of vibrations in the HS state.
To me outstanding theoretical issues concern
- how the HS state softens so many vibrational modes
- defining a simple model effective Hamiltonian that captures the interplay of spin and the vibrational degrees of freedom
- describing and understanding co-operative effects that lead to the first order transition and hysteresis.