An end to a frustrating materials search

The Heisenberg model for anti-ferromagnetically coupled spins on an isotropic triangular lattice is one of the most widely studied (and mentioned) lattice models in quantum many-body physics. This is in spite of the absence of any actual materials described by it! This incredible theoretical interest was stimulated by Anderson's 1987 Science paper on an RVB theory for cuprate superconductors. He invoked his earlier work with Fazekas suggesting that the model had a spin liquid ground state. This turned out to be incorrect: the model does exhibit magnetic ordering and spontaneously broken symmetry, just like conventional antiferromagnets.

However, the materials drought may be over. There is a recent PRL, Experimental Realization of a Spin-1/2 Triangular-Lattice Heisenberg Antiferromagnet
by Yutaka Shirata, Hidekazu Tanaka, Akira Matsuo, and Koichi Kindo

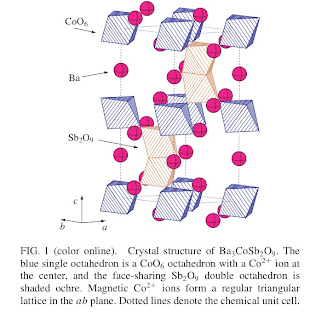
They report magnetisation measurements on Ba3CoSb2O9, and argue that the magnetic Co2+ ion has a spin 1/2 [Kramer's doublet] arising from a combination of crystal field and spin-orbit coupling effects. The temperature and magnetic field dependence of the magnetisation are quantitatively consistent with a spin isotropic Heisenberg model on the triangular lattice with J=18 K and g=3.8. Of particular note, is that they observed a theoretically predicted plateau in the magnetisation, due to a new magnetically ordered phase, which is unstable in the classical model.

These experiments were performed on powders. Hopefully someone will be able to grow large single crystals suitable for inelastic neutron scattering. This enable testing theoretical predictions that due to the interplay of frustration and quantum fluctuations the spin excitation spectrum is quite anomalous. In particular, it should 
exhibit "rotons" which are remnants of RVB physics.


An important theoretical goal will be to derive the effective Hamiltonian and its parameter values from DFT-based electronic structure calculations, as was done recently [and rather nicely] for Cs2CuCl4 and Cs2CuBr4.