A powerful probe for mapping out Fermi surface properties in strongly correlated electron materials

A few years ago Malcolm Kennett and I developed a theoretical formalism that enabled the extraction of information about Fermi surface properties of layered metals from an analysis of the dependence of interlayer magnetoresistance on the direction of the magnetic field. We then contacted Nigel Hussey to get feedback on our paper before we submitted it. It turned out Nigel and his experimental group in Bristol were pursuing similar ideas to extract new information about the temperature and anisotropy (around the intralayer Fermi surface) of the scattering rate in overdoped cuprate superconductors.

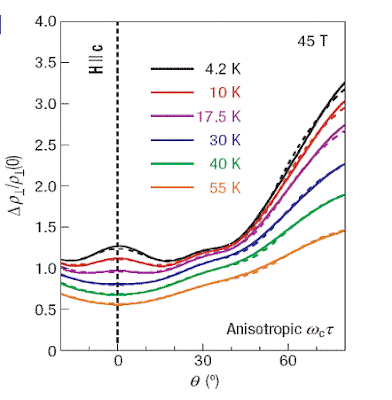
The figure below, from our Nature Physics paper, shows the dependence of the interlayer resistance on theta, the angle between the field and the normal to the layers of the metal. Solid lines are experimental data for an overdoped cuprate superconductor in a magnetic field of 45 tesla and at temperatures varying from 4 K to 55 K. The dashed line are the results of our
theoretical calculation which includes anisotropy in the scattering rate on the Fermi surface. The sample is overdoped, i.e. the superconducting transition temperature Tc=15K is much less than that of optimally doped material which has a Tc=95 K.The Figure below shows the temperature dependence of the anisotropic component of the scattering rate extracted from the theoretical analysis of the experimental data.I found this particularly interesting because the scattering rate showed there were "hot spots" at the same points on the Fermi surface as the nodes in the superconducting gap. This was exactly what was observed with ARPES in underdoped cuprates. However, our finding conflicted with Andrea Damascelli's groups ARPES results on the same material; they found "cold spots" in the overdoped cuprates. This difference has still not been resolved. But, it does underscore the value of this new magnetoresistance probe because it is a bulk measurement and has much higher "energy resolution" than ARPES.

The paper Malcolm and I wrote describing the theory wrote got some pedantic reviews at Physical Review Letters but was eventually published in Physical Review B.
In the next post I will discuss how this technique can also be used to study anisotropies in the pseudogap.