Can strongly correlated electrons save the planet II?

Several earlier posts discussed the thermoelectric effect in strongly correlated electron materials. The Seebeck coefficient S is a quantitative measure of the effect. At low temperatures it can be orders of magnitude larger than in elemental metals. 

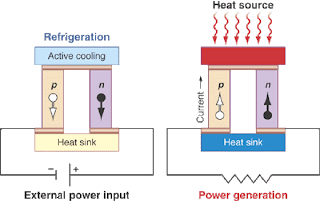
The figure above illustrates how thermoelectric couples can be used to either perform refrigeration or generate electrical power from waste heat. It is taken from a nice Perspective in Science Smaller is Cooler by Brian Sales which reviews state of the art materials in 2002.

The thermoelectric figure of merit, ZT is a dimensionless ratio which is a good measure of how useful a material will be in thermoelectric applications.

sigma is the conductivity and kappa the thermal conductivity.

Currently used materials such as Bi2Te3 [also a topological insulator!] have values of ZT ~1. If materials can be found with ZT~4 then thermoelectric refrigerators will be competitive with traditional compressor refrigerators, which are less reliable and environmentally dirtier.

So how good are strongly correlated electron materials?

There is a nice review by Paschen on heavy fermions.

It is important to note that the thermal conductivity is the sum of electronic and phonon contributions. If one neglects the latter (for the moment) and uses the Wiedemann-Franz ratio then ZT ~ S^2 where S is in units of k_B/e. This is indeed its magnitude near the coherence temperature in strongly correlated electron materials. Hence, ZT ~ 1 (but not larger) seems possible.

BUT, this argument neglects the thermal conductivity due to phonons which is much larger that the electronic contribution in this temperature regime. So one needs to find a way to reduce this. This leads to the idea of a Phonon Glass Electron Crystal.

Candidate strongly correlated materials may be skutterudites which exhibit heavy fermion behaviour (e.g. SmPt4Ge12).