Dielectric relaxation in organic electronic devices

A recent post mentioned the paper Low-k insulators as the choice of dielectrics in Organic Field-Effect Transistors [by Veres et al.], in the context of the sticky point of how to determine the relative importance of disorder and polaronic effects in molecular conducting materials used in semiconductor type devices. 

A major point of the paper is something different: how the mobility measured by time of flight (TOF) measurements is distinctly different [it has a larger magnitude and smaller activation energy] from in FETs, and that the mobility in FETs can decrease significantly with increasing the dielectric constant of the material used in the gate insulator material.

The paper discusses extensively how this may be associated with different kinds of disorder at the interface [which was followed by a theoretical paper in J. Chem. Phys. by Richards, Bird, and Sirringhaus]. It is not clear to me that invoking disorder is necessary to explain the gate dielectric dependence sure this is necessary. The reorganisation energy (polaron binding energy) associated with charge transfer between neighbouring molecules varies significantly with the dielectric constant of the surrounding medium. [Reminder: the activation energy for the mobility is 1/4 of this reorganisation energy].

For molecules close to the interface between the gate insulator and organic semiconductor, this reorganisation energy will decrease with a decrease in the dielectric constant of the insulator. Furthermore, if the organic semiconductor has a dielectric constant less than the gate dielectric, the reorganisation energy associated with a bulk measurement such as TOF will be less than a FET measurement which measures charge transport close to the interface.

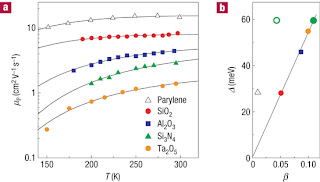
Indeed the observed dependence of the FET mobility on the gate dielectric constant is observed explained within the framework of small polaron theory in a 2005 Nature Materials paper, Tunable Frohlich polarons in organic single-crystal transistors [see Figure above].