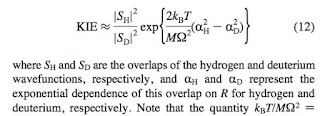An important but basic research skill: not getting too distracted

Making progress in science requires significant focus and discipline. In some sense you need to put in your 10,000 hours. People can distracted by all sorts of non-scientific pursuits: Facebook, romance, family dramas, hobbies, partying, .... But this post is about scientific distractions. I illustrate this will two extreme caricatures.

John really wants to understand his Ph.D project in experimental solid state physics at a deep level. He thinks the quantum measurement problem is really interesting and so he is reading a lot of papers about that. The software he needs for his experiment is functional but he does not like some of the way it interfaces with other software and so he is rewriting it all. In one month he is giving a talk at a conference and so he is not going in the lab for the next month because he wants to give a really nice talk. Whenever his advisor gives him a paper to look at he not only reads it but some of the background references. He spends a lot of time talking to people in a theoretical biophysics group because he thinks what they are doing is pretty interesting....
He is going to struggle to finish his Ph.D in the normal time frame.

Joan is very focussed on her Ph.D project in computational chemistry. She spends most of her time writing and debugging code. She only goes to seminars that she thinks are directly related to her project. The only papers that she has read are those written by her advisor. She rarely talks to students outside her research group. She will finish her Ph.D in a timely manner but may struggle to get a postdoc because she does not have the big picture or an ability to communicate with others outside her narrow area.

So there is balance between the extremes of John and Joan. Too many distractions is bad. But occasionally a "distraction" can be very helpful. I am not sure how to find the balance.

Harden McConnell was a very successful physical chemist. In the last few years of his life he wrote a fascinating scientific autobiography on a web site. In The Young Scientist - My experiences he writes

 My main blunder throughout my career was a kind of scientific introversion – not paying attention to the scientific work of others. As a stellar example, Clyde Hutchison was starting up research on paramagnetic resonance not many steps away from where I sat in the Eckhardt Physics building of the University of Chicago. It’s never too late to look over your shoulder and see what others are doing, and talk to them.

I thank Steve Boxer for bringing Harden McConnell's site to my attention.

Coupled electron-proton transfer: adiabatic or non-adiabatic?

Sharon Hammes-Schiffer gave an interesting talk in Telluride last week about coupled electron-proton transfer.
[A couple of my earlier posts on this fascinating subject are here and here].

Here are a few things that stood out.

There are a lot more people working on this problem now than twenty years ago. This is because of possible solar energy applications.

Diabatic states are the key to understanding. There are four relevant states. Simply the proton can be on the donor or acceptor. The electron can be on the donor or the acceptor. Whether the process is concerted or sequential depends on the relative energy of these four states.

A key question is whether the process is adiabatic or non-adiabatic.
What are the key experimental signatures of each?
One contrast is coupled electron-proton transfer (EPT) and hydrogen atom transfer (HAT).

The two cases are nicely embodied respectively in the model systems
HAT - benzyl/toluene
EPT - phenoxly/phenol
The theoretical details are worked out here.

In some enzymes such as soybean lipoxygenase (SLO) there are very large kinetic isotope effects (~80) for proton transfer, orders of magnitude larger than expected. Many people, including me, have struggled to understand this in terms of proton tunnelling in an adiabatic picture with coupling to an environment. 
However, the relevant reactions are actually coupled electron-proton transfer, in the non-adiabatic regime. The key equation to understand both the magnitude and temperature dependence of the isotope effect is

taken from this paper.

A recent paper compares the theory to a mutant of SLO in which the isotope effect becomes ~500 as a result of the increase in the proton donor-acceptor distance R.

One minor point on how this relates to my talk. I said that quantum nuclear effects [and H/D isotope] effects were largest [and very subtle] in hydrogen bonding for donor-acceptor distances of R= 2.4-2.5 Angstroms. In contrast, here the isotope effects actually get larger with increasing R, with R=2.7 A for the wild-type SLO and increasing to 2.8-2.9 A with the selected mutations. I thank Sharon for pointing out this difference to me.

Quantum biology smells bad

I am skeptical of the grand and speculative claims of "quantum biology". 
There is a nice paper in PNAS which systematically considers the specific claim that smell is based on sensing the vibrational frequencies of particular molecules, and rebuts it from both theoretical and experimental points of view.

Implausibility of the vibrational theory of olfaction
Eric Block, Seogjoo Jang, Hiroaki Matsunami, Sivakumar Sekharan, Bérénice Dethier, Mehmed Z. Ertem, Sivaji Gundala, Yi Pan, Shengju Li, Zhen Li, Stephene N. Lodge, Mehmet Ozbil, Huihong Jiang, Sonia F. Penalba, Victor S. Batista, and Hanyi Zhuang.

I thank Suggy Jang for bringing the paper to my attention.

Triplet superconductivity in a quasi-one-dimensional metal

Last week I was at Stanford and my collaborators and I finished a paper
Spin triplet superconductivity in a weak-coupling Hubbard model for the quasi-one-dimensional compound Li0.9Mo6O17
Weejee Cho, Christian Platt, Ross H. McKenzie, and Srinivas Raghu

The purple bronze Li_0.9Mo_6O_17 is of interest due to its quasi-one-dimensional electronic structure and the possible Luttinger liquid behavior resulting from it. For sufficiently low temperatures, it is a superconductor with a pairing symmetry that is still to be determined.  To shed light on this issue, we analyze a minimal Hubbard model for this material involving four Molybdenum orbitals per unit cell near quarter filling, using asymptotically exact perturbative renormalization group methods. We find that spin triplet odd-parity superconductivity is the dominant instability. Approximate nesting properties of the two quasi-one-dimensional Fermi surfaces enhance certain second-order processes, which play crucial roles in determining the structure of the pairing gap.  Notably, we find that the gap has “accidental nodes”, i.e. it has more sign changes than required by the point-group symmetry.

Earlier relevant posts are:
Weak coupling can give important insights which describes the renormalisation group method used in the paper and results obtained using it.
Desperately seeking triplet superconductors

We welcome comments.
Hopefully the paper will stimulate experiments to definitively determine the nature of the superconducting pairing.

Telluride talk on competing quantum effects

Tomorrow I am giving a talk, "Competing quantum effects in hydrogen bonding: geometric isotope effects and isotope fractionation" at the meeting on Quantum effects in condensed phase systems

Here is the current version of the slides.

The talk is largely based on these two papers

Effect of quantum nuclear motion on hydrogen bonding

Isotopic fractionation in proteins as a measure of hydrogen bond length

Quantum nuclear effects in condensed phase chemistry

I am currently in Telluride for a meeting on Quantum effects in condensed phase systems. Two years ago I attended a similar meeting and in preparing it has been helpful to re-read several posts I wrote stimulated by that meeting.

In my first post, I listed possible quantum effects [zero-point motion, tunnelling, geometric phases, entanglement, ...] and pointed how generally one expects a condensed phase environment [protein, glass, solvent] for a molecular system will tend to reduce these quantum effects by decoherence.

I then asked two big questions.
Are there any instances where the environment can
A. enhance quantum effects?
B. lead to qualitatively new effects (e.g. associated with collective degrees of freedom) that are absent in the gas phase?

I clarified what I meant by a trivial vs. non-trivial enhancement of a quantum effect, from a physics point of view. An example of a "trivial" enhancement is where the environment changes the molecular geometry to enhance the effect. But I stressed that such an enhancement may be highly valuable from a chemistry or biochemistry point of view.

In a comment, Gautam Menon suggested that the Surface Enhanced Raman scattering was a nice example of a non-trivial enhancement. It is certainly spectacular, with enhancements as large as 10^11. However, I am not sure this is the type of quantum effect I am thinking of. The actual mechanism of the effect is still debated [see this paper] and I am not qualified to consider the relative merits of the alternative explanations, but it does look to me like it could be viewed as a semi-classical effect.

Tom Miller suggested to me that the solvation of single electrons and the associated polarons may be a suitable example of B.

I suggested that there were two important organising principles for describing and understanding quantum nuclear effects
1. Competing quantum effects
2. Rate processes can be dominated by rare quantum events.

I am looking forward to the meeting.

Yoichuro Nambu (1921-2015): spontaneously broken symmetry in particle physics

Yoichuro Nambu died earlier this month, and there was an obituary in the New York Times yesterday. He shared the Nobel Prize in Physics in 2008, and is best known for this paper

Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. I 
 Y. Nambu and G. Jona-Lasinio

I reproduce the abstract below because it really does summarise the work and is a nice example of a beautifully written abstract.

It is suggested that the nucleon mass arises largely as a self-energy of some primary fermion field through the same mechanism as the appearance of energy gap in the theory of superconductivity. The idea can be put into a mathematical formulation utilizing a generalized Hartree-Fock approximation which regards real nucleons as quasi-particle excitations. We consider a simplified model of nonlinear four-fermion interaction which allows a γ5-gauge group. An interesting consequence of the symmetry is that there arise automatically pseudoscalar zero-mass bound states of nucleon-antinucleon pair which may be regarded as an idealized pion. In addition, massive bound states of nucleon number zero and two are predicted in a simple approximation.
 The theory contains two parameters which can be explicitly related to observed nucleon mass and the pion-nucleon coupling constant. Some paradoxical aspects of the theory in connection with the γ5 transformation are discussed in detail.

I offer a few minor contextual comments, in order of decreasing significance.

1. Nambu's work is a very nice example of the cross-fertilisation between solid state physics and elementary particle physics. Before Nambu's paper it went mostly one way: solid state theorists used field theoretical techniques. However, Nambu showed how significant new insights in particle physics could be obtained from solid state analogues.

2. Before Nambu there was a lot of concern about the fact that BCS theory was not gauge invariant. He clarified this to the point that these objections were considered dealt with. However, I still get confused about this because of subtle issues about the Goldstone boson [associated with the broken U(1) gauge symmetry of electromagnetism] being "renormalised" by the Coulomb interaction leading to gapped plasmons. Even today there is still debate about whether there is a spontaneously broken symmetry or whether superconductors are topologically ordered, as advocated here.

3. One elegant and technical aspect of this paper was that he introduced the Nambu matrices for describing superconductivity. These and the associated Lie algebras naturally generalise to more complicated situations such field theories and superfluid 3He where the order parameter has 3 spin and 3 orbital degrees of freedom. I found this approach incredibly useful when I did my Ph.D thesis on order parameter collective modes in superfluid 3He-B. Some of this is described here.

4. Was Nambu at the right place at the right time?
 In a previous post, Born for success in quantum many-body theory, I noted how more than half of the founders of the application of field theory techniques to solid state physics were born between 1923 and 1926. Nambu was born in 1921.

Common challenges with constructing diabatic states and tight-binding models

I wish to highlight some common issues that occur in the construction, justification and parametrisation of effective Hamiltonians in both theoretical chemistry and solid state physics.
The basic issue is one needs to keep in mind that just because one gets the energy eigenvalues of a quantum system "correct" does not mean that one necessarily has the correct wave function.
Previously, I posted how sometimes a variational wave function can give  a good ground state energy but be qualitatively incorrect.

For molecular systems a powerful approach to understanding the potential energy surfaces of the ground state and the lowest lying electronic states is to construct a Hamiltonian matrix based on a few diabatic states.

For crystals in which the electronic degrees of freedom are strongly correlated a powerful approach is to construct a Hubbard model where the non-interacting band structure is described by a tight-binding model. The latter describes hopping of electrons between orbitals that are localised on individual lattice sites.

Quantum chemistry
There are two strategies that are used to construct and parametrise a diabatic state model.

1. Based on chemical insight one writes down a Hamiltonian of the form

One assumes some functional form for the Hamiltonian matrix elements, with several free parameters.
One calculates the adiabatic potential energy surfaces using an ab initio method and fits these surfaces to the adiabatic energies from the diabatic model.
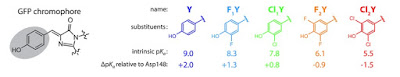
An example is shown below for a two-state diabatic model for fluorescent protein chromophores.

The  example below concerns five electronic states of XH3 and the associated torsional potential, taken from this paper. There are 11 free parameters in the Hamiltonian.

A different approach by Nangia and Truhlar considers a multi-dimensional potential surface for ammonia, two diabatic states, and hundreds of free parameters.

Important questions arise.
Are the diabatic states physical?
Or is all this curve fitting just making the elephants trunk to wiggle? 
As one includes more diabatic states how does one deal with the confusion and ambiguity that arises because of the close proximity to one another of many excited states?

2. A more rigorous approach is to use some well-defined procedure to actually construct the diabatic states from a knowledge of the many-body wave functions of the low lying states. An example is the approach pioneered by Cederbaum. Seth Olsen has nicely used this approach to construct diabatic states for fluorescent protein chromophores and other organic dyes. Furthermore, the diabatic states can be related to chemically intuitive valence bond structures.
However, subtle issues still arise, particularly as one includes more excited states.

Solid state physics
Similarly, there are two strategies that are used to construct and parametrise a tight-binding model for a specific material.

1. One writes down a tight-binding model Hamiltonian with a few parameters describing hopping integrals and calculates the associated band structure. One then calculates the band structure for a specific material, using an ab initio method, usually some approximation of Density Functional Theory (DFT). One then fits this band structure to the tight-binding model in order to determine the hopping integrals.

An example is shown below, taken from this paper. The green dots are from an DFT based calculation and the solid black lines are a fit to a tight-binding model with a few free parameters.

This procedure gets messy and ambiguous when in order to improve the quality of the fit one starts to introduce extra parameters representing beyond next-nearest neighbour hopping. 

Are such long range hoppings justified? Furthermore, the parameter values obtained can vary significantly as one introduces extra parameters.

2. A more rigorous approach is to construct Wannier orbitals and then calculate the actual overlap integrals that are input into a tight-binding model.
An example is in this paper concerning the Fabre salts. In particular it shows how some longer range hoppings are actually justified.
However, there are many subtleties and ambiguities in this approach as discussed in a recent Reviews of Modern Physics. This tends to work well when there are a couple of well isolated bands, but not otherwise.

Clearly, 2. is always preferable because it has a stronger physical basis. However, it is not easy. People tend to be just do 1. with a fixed number of parameters and not worry about whether they are justified or stable.

I thank Seth Olsen and Anthony Jacko for teaching me about these issues.

Tips on preparing an application for a multi-disciplinary review panel

If you applying for a grant or a fellowship that involves competing with people from other disciplines consider the suggestions below. Most of these applications are reviewed and decided by committees that comprise people from a range of disciplines, e.g. from marine biology to theoretical physics.

Remember your audience
They are mostly not in your field and they are busy and jaded. They will look over [n..b I did not say read] your application very quickly.

Count the cost of applying
These applications are actually much more work than field focused [e.g. condensed matter theory] ones. Don't kid yourself you can just cut and paste material from other applications. Don't kid your self that your "excellent" "track record" and "outstanding" "research project" will carry the day. Inevitably, you will be competing with a few individuals who will spend a lot of time carefully crafting a compelling application that does things like those below.

Kill the jargon
Not only can't non-experts understand it they may even resent your for it and rank you lower.

Less is more
Cut material out. Try and get over a few simple details and achievements.
Avoid long lists. For example, rather than a list of 10 invited conference talks, say you had 10 and give details about 3 saying why those are particularly important conferences.
Figures help.

Provide a clear and concise statements of your major scholarly achievements.
This is what I most desperately seek in applications, yet often don't find.
For example, if asked to list your 5 most significant publications, provide a 30 word statement
for each stating why it is significant.

Provide context for everything
[significance of project, journals, author order, citations, grants, prizes]
Outsiders don't know. You have to tell them.
For example, saying you were a finalist in the APS Leo Apker award won't mean anything and its significance will be lost. Non-mathematicians need to be told that most author lists are alphabetical and publication and citation rates in mathematics are much lower than in other fields.

Don't under-estimate the power of the joy of learning
I have noticed that even colleagues who suffer from metric madness are favourably disposed when they actually learn something new about a different field.
on the flip side, if your application makes people feel dumb that will work against you.

Diabatic states rock!

Physical Chemistry Chemical Physics has just published a series of four articles by Jeff Reimers, Laura McKemmish, Noel Hush, and myself.

A unified diabatic description for electron transfer reactions, isomerization reactions, proton transfer reactions, and aromaticity"

Non-adiabatic effects in thermochemistry, spectroscopy and kinetics: the general importance of all three Born-Oppenheimer breakdown corrections

Electron-vibration entanglement in the Born-Oppenheimer description of chemical reactions and spectroscopy

Bond angle variations in XH3 [X=N,P,As,Sb,Bi]: the critical role of Rydberg orbitals exposed using a diabatic state model

It took a number of years to finish these papers. I am certainly the junior co-author and I commend my co-authors for all their hard work and perseverance.

The four papers have two common related themes, that are hopefully not lost in all the technical detail.

1. Diabatic states provide a powerful scheme, both conceptually and quantitatively, to describe a wide range of chemical phenomena.
2. This can be nicely illustrated using a simple model Hamiltonian describing the coupling of two electronic states to a single vibrational mode.
In chemistry language this is a E x beta Jahn-Teller model. In condensed matter language, is a two-site spinless fermion Holstein model.

We welcome comments.

Update (24 September, 2015). The papers made it to the cover of the print edition.

What is a good publication rate?

I really think this is the wrong question.
I increasingly get irritated by grant reviewers passing judgement on people, particularly junior scientists, because their "publication rate" is "not high enough" or "good but not outstanding".

First, we should be concerned with the quality of the publications; i.e. the significance, originality, technical difficulty, and reliability of the scientific content. Quality is more important than quantity. A major problem with science today is not that people are not publishing enough papers! Rather a problem is the low quality of what is produced. If you look at Einstein, Feynman, Onsager, .... their publication rates were not very high. Furthermore, even lesser mortals such as Mermin, Hohenberg, Haldane, ... have publication rates, particularly early in their career, that might be rated as "good but not outstanding" by some grant assessors today.

Second, given most papers are multi-author one should be concerned with the quality and quantity of the individuals contribution to each paper. Just dividing number of papers / number of authors is not good enough.

Third, one needs to factor in opportunity. The publication rate of a junior faculty member with little funding and a heavy teaching load should not be compared to a well funded senior faculty member who does no teaching.

Do I look at publication rates of grant applicants and job candidates? Somewhat, but only as a secondary measure, and in context. I do like to see a somewhat "steady" output of a "few" papers per year in "decent" journals. I do sometimes get concerned about people who seem to produce "little" relative to opportunity. But, what I am really concerned about is whether the person is producing some useful and interesting scientific knowledge.

Finally, given all this silliness let me encourage you to try and keep your publication rate up by using publons and choosing to work with organised people who are good at getting papers "out the door", but with integrity.

Genetically engineering short hydrogen bonds in a fluorescent protein

There is a very nice article in the new journal, ACS Central Science
Short Hydrogen Bonds and Proton Delocalization in Green Fluorescent Protein (GFP) 
Luke M. Oltrogge and Steven G. Boxer

This is an impressive piece of work spanning from molecular biology to chemistry to quantum physics.
There is also a commentary on the paper by Judith Klinman, placing it in the context of the controversial issue of low-barrier hydrogen bonds in enzymes.

An extensive study was made of mutants of the Green Fluorescent Protein with a short hydrogen bond between the chromophore and the amino acid Asp148. The donor-acceptor bond length estimated from X-ray structures was 2.4 +/- 0.2 Angstroms. This is in the range of low-barrier H-bonds.

What is particularly new here is that through ingenious molecular biology techniques [nonsense suppression] the acidity [pK_a = measure of tendency to give up protons] of the chromophore was systematically varied by 3.5 units through halogen substitutions.

This range covers the pK_a matching required for strongest H-bonds, as discussed in this earlier post. The experimental results were compared to calculations based on a one-dimensional proton transfer potential based on a diabatic state model I have advocated. It was very satisfying for me to see this simple model being used by experimentalists.

To me what is most striking about the paper is the UV absorption spectra below. It is very different from what one normally sees in GFP spectra.

There are generally two absorption bands, denoted A and B, associated with GFP. The A-state and B-state are identified with the neutral chromophore and anionic [i.e. deprotonated] chromophore, respectively. The corresponding spectra are similar to the black and grey curves shown above. The green spectrum above is for the Cl1Y substituted chromophore, which is close to pK_a matching, and is rather broad and intermediate between the A-state and B-state spectra. This is arguably because the proton is delocalised between the chromophore and neighbouring Asp amino acid.

The authors also substituted protons (H) with deuterium (D) to see the extent of quantum nuclear effects. These are normally very small in GFP. However, here they are noticeable.
The measured isotopic fractionation factors Phi (deduced from analysis of the UV absorption spectra) were in the range 0.54 - 0.9, taking a minimum value for pK_a matching. This observation and a value of Phi=0.54 for R=2.4 +/- 0.2 Angstroms are consistent with a recent theoretical analysis.

There is one point where I disagree with the theoretical analysis of the authors. I am confused that they average over the vibrational eigenstates to get an electronic absorption spectrum. This seems to me this goes against the Franck-Condon principle.  If one followed this same procedure for other molecules the UV spectra would all be much broader than they are, particularly in gas phase.
It is not clear to me how one should proceed in this situation where the proton is quite delocalised and the absorption spectra is significantly different for protonated and de-protonated chromophores. There may be significant Herzberg-Teller effects. One way forward to could be to combine the two-diabatic state H-bond model with a two-state resonance model for the chromophore, such as those advocated by Seth Olsen and I, and then do a full non-adiabatic treatment of the model.

I thank Luke Oltrogge and Seth Olsen for helpful discussions about this work.

Do you want to be judged at the click of a mouse?

Then sign up for a Google Scholar account!

With one click people will not just see your publications but also how many times they have been cited. More problematic is that they will also see the values of different metrics such as your h-index and ten year h-index.

Recently, I encouraged someone on the job market to delete their account.
Why?

Unfortunately, there are people who will look at job and funding candidates and quickly dismiss them  if their metrics fall below certain threshold values. No consideration is given to scientific content, quality of publications, difficulty or popularity of the research field, career or personal history, .... People inevitably make unhealthy and unrealistic comparisons to "stars" and more senior people.
I have seen this happen.

I detest this and so I do not have my own account. Furthermore, I do not look at peoples pages just for the sake of it. I particularly think making comparisons with colleagues is very unhelpful.

So here are my reluctant and painful recommendations which some will disagree with.
Basically, unless you have "stellar" citations I would delete your page or not get one.
What are some rough numbers for h-index cutoffs? I would suggest.
Ph.D students should not have an account.
If you are a postdoc anything in single digits.
If you are junior faculty anything less than 20.
If you are senior faculty anything less than 30-40.

I stress I do not agree with this. I am just trying to protect you.
In an actual written application you can provide whatever citation information you choose. Furthermore, you have the opportunity to actually spell out your real scientific achievements and put your career in context.
Don't provide lazy evaluators with an easy option.

I welcome comments.

A nice write up in Physics World

Physics World [Magazine for the Institute of Physics (UK) = British equivalent of Physics Today] has a feature Web life that covers different web sites.

The June 2015 issue features this blog!
Besides the publicity, I was really happy because I felt the article nicely captured what I am on about. I was not interviewed and only heard about it from a Commenter, Peter Morgan.

One mild amusement was that I was classified as "a chemical physicist". I would certainly classify myself as a "condensed matter physicist" who sometimes tries to do chemical physics. So I took this as a compliment!