2013 Nobel Prize in Chemistry: Where is the quantum-classical boundary?

This is relevant to the 2013 Nobel Prize in Chemistry, awarded to Martin Karplus, Michael Levitt, and Arieh Warshel, for "Development of multiscale models for complex chemical systems."

This question means somewhat different things to physicists and chemists. To physicists it means "how big does a system have to get for it start behaving in a classical manner?"
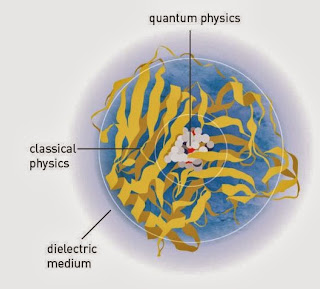
To chemists it means "where can I draw a spatial boundary between the part of the system of interest that I want to treat quantum mechanical and the part I will treat classically.
This is illustrated in the Figure below, taken from the official "Scientific background for the prize."
It is worth reading.

This partition of the system is central to the tutorial, "Effective Hamiltonians for quantum dynamics in functional molecular materials," I gave on monday.

To be honest I have some mixed feelings about this prize. First, I wonder if the citation should read, "Development of multiscale computational modelling techniques for complex chemical systems." To me "a model" and "computational modelling" are quite different things. Although, perhaps the point is that "a model Hamiltonian" is at the heart of the simulations.

On the one hand, the recipients have all made monumental contributions to an incredibly difficult and important problem. They have stimulated a whole new research field, for better or for worse. But, to some the nagging question remains as to how robust and useful these simulations are. What new chemical insights do they give? In some cases, they have been successfully used to elucidate reaction mechanisms and rule out alternatives. I fear the answer is that the simulations are very useful in the hands of Karplus, Levitt, Warshel, and a few others. In the hands of the masses they may be just misleading and dangerous.

In particular, are the simulations falsifiable? A good outcome for science will be if the Prize stimulates renewed efforts to attack the fundamental scientific problems that these simulations aim to address. A bad outcome would be if more money is just spent hiring people to run existing codes to "simulate" more complex systems.

What do you think?