The shear viscosity can be written in terms of a Kubo formula which is an unequal time correlation function of the stress energy tensor.
In a general fluid there are two terms in the stress energy tensor: one associated with the kinetic energy and the second with the interparticle interaction. In dense classical liquids the term in the Kubo formula due to the interaction term dominates and are associated with the Einstein-Stokes relation where the viscosity is inversely proportional to the particle self-diffusion constant.
In contrast, in dilute gases and fluids the kinetic term dominates and the shear viscosity scales with the diffusion constant and scattering time. The crossover from the dilute to the dense case in a classical fluid is discussed here.
The case of the dilute classical gas is of particular historical interest. The viscosity scales with the density and the mean-free path. In a dilute gas the mean free path is inversely proportional to the density and the molecular cross section. This means that the viscosity is independent of the density (and pressure at fixed temperature). When Maxwell obtained this theoretical result from kinetic theory he found it so surprising that he tested it experimentally. According to this site,
In the attic of his house in Kensington, with the help of his wife, he carried out experimental measurements of gas viscosities in order to confirm the conclusions he had drawn about the effects of pressure and temperature. Many of these experiments were made between 51 °F (10.6 °C) and 74 °F (23.3 °C), and it appears that these temperatures were obtained simply by changing the temperature of the attic! This was arranged by Mrs. Maxwell, who organized the appropriate stoking of the fire. Some work was also done at 185 °F (85 °C), and this temperature was achieved by a suitably directed current of steam.The results are described in this 1866 paper.
For a zero-range interaction, as in the unitary Fermi gas (and presumably the Hubbard model), it can be shown that the potential term does not contribute to the shear viscosity. For a succinct discussion of these issues and relevant references see the section of this paper that I reproduce below. I thank Thomas Schafer for pointing this out to me.
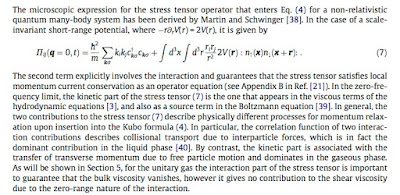



"For a zero-range interaction, as in the unitary Fermi gas (and presumably the Hubbard model), it can be shown that the potential term does not contribute to the shear viscosity"
ReplyDeleteTo be clear, you mean that the potential term doesn't contribute to the stress tensor; it most certainly contributes to the shear viscosity via the expectation value of the stress tensor correlation function.
Hi Edward,
DeleteThanks for the expert comment.
But, I am confused.
The last sentence of the paragraph I reproduce says, "for the unitary gas the interaction part of the stress tensor .... gives no contribution to the shear viscosity due to the zero-range nature of the interaction."
Could you please clarify?
thanks
Ross
This is just a matter of wording. The authors point out (correctly) that the interaction part of the stress tensor doesn't contribute to the shear viscosity. As in, it does not enter the Kubo formula that determines the viscosity from the stress-stress correlator.
ReplyDeleteThis is very different from saying that the potential term doesn't contribute to the shear viscosity which I took to mean that tuning the interaction energy (which of course can be done in dilute alkali Fermi gases) has no effect on the shear viscosity. The shear viscosity of an ideal gas is infinite; turning on interactions it becomes finite and increasingly small as the interaction is "turned up". In quantum gases, it reaches a minimum close to unitarity, on the order of density times hbar.
Both the shear and bulk viscosities also admit Kubo formula expressions in terms of the current-current correlation functions, which I find to be an easier way of thinking about them. Here, as with e.g. the conductivity of an electron gas, the current operator contains no explicit dependence on the interaction, yet the expectation value of its correlation function of course depends on its strength. In contrast, because it in general depends explicitly on the interaction potential, the stress tensor operator can be unwieldy to use (although useful for AdS-CFT calculations because it results from the deformation of the action with respect to the metric in curved space-time).