Excited state potential energy surfaces for organic dyes

Sean McConnell, Seth Olsen, and I just finished a paper
A Valence-Bond Nonequilibrium Solvation Model for a Twisting Cyanine Dye

We study a two-state valence-bond electronic Hamiltonian model of non-equilibrium solvation during the excited-state twisting reaction of monomethine cyanines. These dyes are of interest because of the strong environment-dependent enhancement of their fluorescence quantum yield that results from suppression of competing non-radiative decay via twisted internal charge-transfer (TICT) states. For monomethine cyanines, where the ground state is a superposition of structures with different bond and charge localization, there are two twisting pathways with different charge localization in the excited state. The Hamiltonian designed to be as simple as possible consistent with a few well-enumerated assumptions. It is defined by three parameters and is a function of two π-bond twisting angle coordinates and a single solvation coordinate. For parameters corresponding to symmetric monomethines, there are two low-energy twisting channels on the excited-state surface that lead to a manifold of twisted intramolecular charge-transfer (TICT) states. For typical monomethines, twisting on the excited state will occur with small or no barrier. We show that changes in the solvation configuration can differentially stabilize TICT states in channels corresponding to different bonds, and that the position of a conical intersection between adiabatic states moves in response to solvent to stabilize either one channel or the other. We show that there is a conical intersection seam that grows along the bottom of the excited-state potential with increasing solvent polarity. For solvents of even moderate polarity, we predict that the intersection seam should completely span the bottom of the excited-state potential in these systems.

We welcome any comments.

A unified phase diagram for tetrahedral liquids

At the NORDITA water meeting Charusita Chakravarty gave a nice talk that featured the phase diagram below.

The figure is taken from a nice Perspective paper in PhysChemChemPhys.
Water and water-like liquids: relationships between structure, entropy and mobility 
Divya Nayara and Charusita Chakravarty

The article gives a nice overview, putting the anomalous properties of water in a broad context, comparing and contrasting to the properties of other liquids for which tetrahedral interactions are dominant. Possible relations between thermodynamics, transport, and structure are also discussed.

Key anomalous properties of water [compared to simple isotropic liquids] include
-the negative slope of the melting line in the temperature-pressure phase diagram
-the temperature of maximum density [277.15 K at 1 atm]
-increase in diffusion with increasing density
-increase in specific heat, thermal expansion, compressibility upon isobaric supercooling.

Water is actually not as unique as I thought. Other tetrahedral liquids exhibit similar anomalies. Furthermore, it is not the hydrogen bonding (per se) that makes water anomalous, but rather the tetrahedral interactions associated with the hydrogen bonding.

The figure above is based on the Stillinger-Weber model, a coarse-grained model that captures the competition between two-body interactions and three-body (tetrahedral) interactions. The version of the model for water is termed monatomic Water (mW) and is described in this previous post. At the meeting Jibao Lu described recent work which gave an objective scoring of the successes and failures of different mW models and atomistic models.

Jacob's ladder is not the best Biblical metaphor for computational materials science

A more appropriate metaphor is Jacob wrestling with the angel (God).
This point was made in a nice talk that Mike Gillan gave last week at the NORDITA water meeting.

John Perdew has invoked the metaphor of Jacob's ladder to describe his "dreams of a final theory" and the quest for an "exact" exchange correlation functional for Density Functional Theory (DFT).
Perdew's metaphor was earlier reinvoked by Joost VandeVondele in his talk at the meeting.

This painting of Jacob's ladder is by Michael Willmann. In the Biblical account from Genesis 28

Jacob left Beersheba, and went toward Haran. He came to the place and stayed there that night, ....  And he dreamed, and behold, there was a ladder set up on the earth, and the top of it reached to heaven; and behold, the angels of God were ascending and descending on it! And behold, the Lord stood above it [or "beside him"] and said, "I am the Lord, the God of Abraham your father and the God of Isaac; the land on which you lie I will give to you and to your descendants; and your descendants shall be like the dust of the earth, and you shall spread abroad to the west and to the east and to the north and to the south; and by you and your descendants shall all the families of the earth bless themselves. Behold, I am with you and will keep you wherever you go, and will bring you back to this land; for I will not leave you until I have done that of which I have spoken to you." Then Jacob awoke from his sleep and said, "Surely the Lord is in this place; and I did not know it." And he was afraid, and said, "This is none other than the house of God, and this is the gate of heaven.

This was a dream.
The "ladder" of approximations is also a dream because it conveys the idea that with each rung of the ladder one is necessarily getting closer to the correct answer [heaven]. Things are not that simple. For example, Mike Gillan pointed out to me that the generalised gradient approximation (GGA) does worse than the local density approximation (LDA) for the surface energies of solids.   No doubt experts can provide other examples.

Similar issues arise in wave function based computational quantum chemistry. I think Pople first drew a diagram such as the one below (taken from this paper). The idea is that as one increases the size of basis set and the level of theory (i.e. treatment of electron correlation) one moves closer to reality (experiment).

Again, the problem is that the "convergence" to reality is not monotonic or uniform. This is reflected in the existence of Pauling points. Sometimes as one moves down or to the right one on the figure actually gets further away from the experimental value. This is discussed in detail for a specific example in a paper by Seth Olsen.

As Mike Gillan suggested a more appropriate Biblical metaphor than Jacob's ladder is the account in Genesis 32 of Jacob [whose name means deceiver] wrestling with an angel. Afterwards, he is renamed Israel [which means he who wrestles with God].

The painting is by Rembrandt (1659).

Computational materials science is a struggle. Jacob's ladder is a dream.

Keep repeating your message

Sometimes in life we get irritated at people who keep saying the same thing again and again.
However, I think if you have an important scientific message you need to realise that you may need to keep repeating it. If you have something original and/or profound to say it is not going to be easy for people to grasp and/or accept. With some talks I have no idea what the speaker is trying to say. Talks that end with 10 conclusions don't help! Even for good speakers I find I benefit from hearing the talk several times in different forums over a period of time, interspersed with looking at the relevant papers, and sometimes blogging about them.
I am not alone. I have noticed that even after someone has heard one of my talks several times in different forums and I have talked informally with them about it, there are basic points they still don't appreciate or get on the third hearing.

Action point: don't be shy about giving a similar talk to one you have given before. Try to have just one message, not several. Talking about several topics almost never works.

Similar issues are relevant with teaching. Just telling students something once usually has no impact. They need to hear it several times. Furthermore, encountering the same idea from different sources and through different media may be necessary for the idea, concept, or technique to be understood, appreciated, and mastered. Different media include lectures, tutorials, text books, computer simulations, quizzes, and peer instruction. Furthermore, having different people explain something in different ways can be quite helpful. This is one reason when I teach a class I often review material that someone else may have already "taught" them. This is not because I think I am a better teacher than my colleague. I think students benefit from hearing the same material from someone else and review can be beneficial. I do this because students tell me it is helpful.

Water: anomalies, challenges, and controversies

I really enjoyed this week's meeting Water: the most anomalous liquid at NORDITA. This is the first time I have ever been to a workshop or conference that is solely about water. Here are some impressions and a few things I learnt as a newcomer to the field.

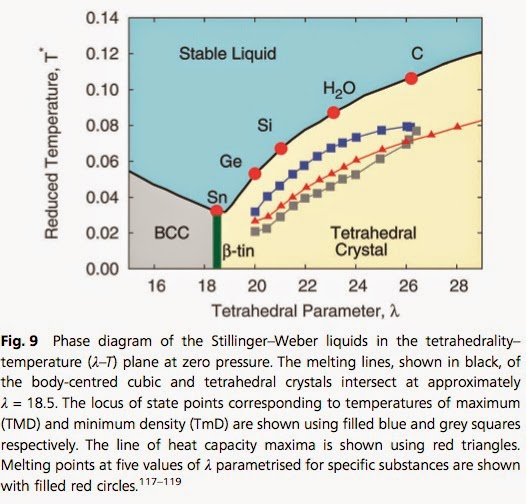
Just how unique and anomalous is water?
Not as unique as I thought. Some other tetrahedral liquids have similar properties.

Hydrogen bonding is not what makes water unique
Rather it is the tetrahedral character of the intermolecular interactions that arise from hydrogen bonding. This distinction can be seen from the fact that the mW (monatomic water) model captures many of the unusual properties of water.

DFT is a nightmare
I have written a number of posts that express caution/concern/alarm/skepticism about attempts to use Density functional theory (DFT) to describe properties of complex materials. Trying to use it to calculate properties of a liquid water in thermal equilibrium is particularly adventurous/ambitious/reckless. First, there is the basic question: can it even get the properties of a water dimer in the gas phase correct? But, even if you choose a functional and basis set so you get something reasonable for a dimer there is another level of complexity/fakery/danger associated with "converging" a molecular dynamics simulation with DFT producing the Born-Oppenheimer surface. This was highlighted by several speakers. Simulations need to give error bars!

A physically realistic force field (at last!)
A plethora of force fields [TIP3P, SPC/E, TIP4P/2005, ST2, ....] have been developed for classical molecular dynamic simulations. They are largely based on electrostatic considerations and involve many parameters. The latter are chosen in order to best fit a selection of experimental properties [melting temperature, temperature of maximum density, pair correlation function, dielectric constant, ....]. Some models use different force fields for ice and liquid water. On the positive side it is impressive how some of these models can capture qualitative features of the phase diagram including different ice phases and give a number of experimental properties within a factor of two. On the negative side: they involve many parameters, it is hard to justify including some "forces" and not others, and give very poor values for some experimental observables [e.g. TIP3P has ice melting at 146 K!]. How often do people get the right answer for the wrong reason?

An alternative strategy is to actually calculate an ab initio force field using state of the art quantum chemistry and a many-body expansion that includes not just two-body interactions (i.e. forces between pairs of molecules) but three-body and beyond interactions. This was discussed by Sotiris Xantheas and Francesco Paesani. An end result is MB-pol.

Quantum zero-point energy is (not) important
Sotiris Xantheas emphasised that semi-empirical force fields are effective Hamiltonians that implicitly include quantum nuclear effects at some effective classical potential [e.g. a la Feynman-Hibbs]. Thus, if one then does a path integral simulation using one of these force fields one is  "double counting" the quantum nuclear effects at some level. Xantheas and Paesani also emphasised that MB-pol should not be expected to agree with experiment unless nuclear quantum effects are included.
On the other hand, due to competing quantum effects classical simulations for water give better results than one might expect.

The elusive liquid-liquid critical point
Some of this controversy reminded me of high-Tc cuprate superconductors where the elusive quantum critical point [under the superconducting dome?] may (or may not) exist. It is also interesting that there is a proposal of a Widom line in the cuprates, perhaps inspired by water.
Some of the arguments and sociology seemed like the cuprates. There are true believers and non-believers. Each camp interprets (and criticises) complicated and ambiguous experimental results and large computer simulations according to their prior beliefs. Kauzmann's maxim is relevant: people will often believe what they want to believe rather than what the evidence before them suggests they should believe.

Perhaps this critical point does not appear in the physical phase diagram of bulk water but can be accessed via "negative pressure" in some force field models. A key observable to calculate is the heat capacity, experimentally it appears to diverge. But its calculation will require inclusion of nuclear quantum effects. [It is not clear to me why you can't just input the classical vibrational spectrum into a non-interacting quantum partition function.]

I felt this issue dominated some discussions at the meeting too much.

The O-O radial distribution function is over-emphasised
In any liquid this pair correlation function is an important observable that is a measure of the amount of structure in the liquid. For water the O-O radial function has been "accurately" measured and provides a benchmark for theories. Getting it correct is a necessary but not a sufficient condition for having a correct theory. But water is an anisotropic molecular liquid not a Lennard-Jones monatomic fluid. Angular correlations are very important for water. Also, unfortunately, other pair correlation functions such as the O-H and H-H radial distribution functions are not well characterised experimentally.

When are the many-body effects quantum?
One can make many-body expansions in electrostatics, classical statistical mechanics, and quantum many-body theory. A profound question is: are there situations, criteria, or properties that can make the latter distinctly different from the former?

Talk on nuclear quantum effects in water

On thursday I am giving a talk "Quantum nuclear effects on hydrogen bonding in water" at the Nordita workshop, "Water: the most anomalous liquid". Here are the slides. It is mostly based on this paper.

Classifying quantum effects in water

This week I am in Stockholm at a NORDITA workshop, Water: the most anomalous liquid.
I am in a working group on Quantum effects in water. The workshop runs for 4 weeks. There will be about 12 working groups. Each is meant to produce a ten page review that will be then be combined into a review article, co-authored by all the participants.

Today we discussed a possible classification of different quantum effects.
They are manifested in H/D [hydrogen/deuterium] isotope substitution experiments.
For equilibrium properties these isotope effects would be non-existent if the nuclear dynamics is treated classically. This is because at the level of the Born-Oppenheimer approximation the potential energy surface for H and D is identical.
For dynamical properties such as the water self-diffusion constant there is a trivial classical effect from the scaling of vibrational frequencies with H/D substitution.

As I mentioned before, most quantum nuclear effects are associated with vibrational zero-point energy. But, there are effects associated with tunnelling and quantum delocalisation such as a in high pressure phases of ice such as ice X.
Here is one possible classification.

Trivial effects.
These arise simply because the H/D substitution changes vibrational frequencies by a scaling factor of sqrt(2)=1.414. An example, is the large difference between the specific heat of heavy and regular water. This simply arises because the thermal population of the vibrational excited states changes because of the change in hbar omega/k_B T. One would observe such a change in almost any solid or liquid.

Significant or non-trivial effects.
Examples are the pH of heavy water, and liquid-vapour isotopic fractionation ratio. The non-trivial dependence of this on temperature [taken from this paper] is shown below. It is intimately connected with competing quantum effects.

Anomalous effects.
These have the opposite sign to what one expects and sees in simple solids and liquids. For example,
the volume expansion from solid H20 and D2O, is the opposite to the contraction that occurs in most solids, as described here.

It would nice to make these classifications a bit sharper.

Tips in the writing struggle

How does one stay sane as one struggles to produce talks, lectures, grant applications, and papers?
Is there some efficient methodology?

I am in the midst of a period of several weeks where I have to give about a dozen talks/lectures. I also have several papers being finished. Hence, this issue is on my mind. Here are a few thoughts that hopefully are helpful to others. I realise that different people have different styles.

I think there are several major obstacles to producing material in a timely manner with a minimum of stress: perfectionism, procrastination, loose ends, and distractions. These are somewhat related to one another.

First, get started a quickly and early as possible. Just get something down on paper. A rough outline is a good place to start. Don't censor your thoughts or agonise about details. Just do it!

Then start adding material, editing, and polishing.
But, spend a limited amount of time. This will mean having the self control to not include that figure, reference, or extra result, alternative derivation, that you would really like to but is going to require some time to track down. This is were perfectionists go astray....
I also find I can easily get distracted by new ideas or new information that is fascinating to me personally but marginally relevant to the task at hand.

Once you have the bare minimum of material, try and produce a version of the document [or power point slides] that is good enough for public consumption/presentation. Imagine that you have to give the talk today not tomorrow or submit the grant 2 weeks early. Then you can sleep on it, give it to colleagues for feedback, or perhaps allow for the reality that you need to move onto other responsibilities or allow for some unforeseen problem like getting sick or dealing with a family or administrative crisis.
I find meeting these artificial deadlines early makes more me much more relaxed and objective. Otherwise one is furiously working up until the last minute.

Then as time allows this final draft can be added to and polished.

I hope this is helpful. It is a struggle.

Kelvin formula for thermopower in bad metals

Jure Kokalj and I just finished a paper,
Enhancement of the thermoelectric power by electronic correlations in bad metals: a study of the Kelvin formula 

In many strongly correlated electron metals the thermoelectric power has a non-monotonic temperature dependence and values that are orders of magnitude larger than for elemental metals. Kelvin proposed a particularly simple expression for the thermopower in terms of the temperature dependence of the chemical potential. We consider a Hubbard model on an anisotropic triangular lattice at half filling, a minimal effective Hamiltonian for several classes of organic charge transfer salts. The finite temperature Lanczos method is used to calculate the temperature dependence of the thermopower using the Kelvin formula. We find that electronic correlations significantly enhance the magnitude of the thermopower and lead to a non-monotonic temperature dependence. The latter reflects a crossover with increasing temperature from a Fermi liquid to a bad metal. Although, the Kelvin formula gives a semi-quantitative description of some experimental results it cannot describe the directional dependence of the sign of the thermopower in some materials.

Jim Brooks (1944-2014): pioneer in high magnetic fields

I was saddened to hear of the recent sudden death of Jim Brooks. He is the experimentalist who arguably has had the biggest impact on me scientifically and my career.

Jim grew up in Los Alamos in an extended family of physicists. He did a Ph.D at U. Oregon with Russell Donnelly as an advisor, working on low temperature physics.
I believe he may have been the first person to put a dilution fridge in a high field [30 tesla] magnet, while working at Boston University and the Bitter Magnet Lab at MIT. This was significant following the discovery of the fractional quantum Hall effect by Tsui and Stormer. After a sabbatical at Princeton with Paul Chaikin [involving the discovery of a quantum Hall state in the field induced spin density wave of a Bechgaard salt] he began to work almost exclusively on organic charge transfer salts. He made many studies that mapped out their rich phase diagrams [as a function of temperature, pressure, uniaxial stress, magnetic field, and chemical substitution] and "fermiology". The latter involved using high magnetic fields and low temperatures to use quantum oscillations [Shubnikov de Haas and de Haas van Alphen] and angle-dependent magnetoresistance oscillations [AMRO] to map out Fermi surfaces.

I first met Brooks in 1994 at a conference in Korea, just after I had moved to University of New South Wales. Later that year he came to UNSW to use the pulsed magnetic field lab, set up by Bob Clark, to perform a series or experiments on organic charge transfer salts, in fields up to 50 tesla. This led to us writing about half a dozen papers together. From 1995 to 2002 he hosted an (approximately) annual visit I made to the Florida magnetic lab. I benefited greatly from these visits.

The most significant scientific thing Brooks did for me was introduce me to organic charge transfer salts and to AMRO. This led directly to some of my best scientific work, such as a review on organics and showing that a 3-dimensional Fermi surface is not necessary for AMRO. My positive experience from talking (a lot) to Brooks heavily flavours the thoughts in my post on listening to experimentalists.

Several times Brooks wrote letters of reference for me that I think were probably very important in my survival/success in science.

Brooks was fun to work with and to be around. He was a bit of a clown. He really did not take himself very seriously, despite his professional stature. The first day he came into the lab at UNSW he arrived on roller blades with all his shirt buttons undone. I remember on one visit to Florida he had dinner with my family, when my kids were very young.  Brooks came out of the bathroom with strings of toilet paper stuffed into his nose! The kids loved it.

On the National High Magnetic Field Laboratory web site there are some nice tributes from a range of people. Brooks biggest legacy is probably the many young people he mentored and supported.

A promising alternative to Dynamical Mean-Field Theory?

At the cake meeting this week we discussed
Density Matrix Embedding: A Simple Alternative to Dynamical Mean-Field Theory 
Gerald Knizia and Garnet Kin-Lic Chan

This is an original and promising approach. It is computationally much "cheaper" than DMFT.

There are follow up papers that apply the method to quantum chemistry, the problem of defining the QM/MM boundary, the honeycomb lattice Hubbard model, and calculation of spectral functions. In the latter the bath is frequency dependent.

The system is divided into an "impurity" and a "bath".
The starting point is the Schmidt decomposition of the system quantum state. If M is the dimension of the impurity Hilbert space then there are at most M terms in the decomposition. This limits the amount of entanglement between the impurity and the bath.

Consider a Hubbard model where the impurity is a single site, then M=4.
The reduced Hamiltonian acting on the Schmidt basis states looks like a two-site Hubbard model, which is easy to solve.
[It is not clear why nearest neighbour Coulomb repulsion or more complicated 4-body terms are not included].
Expectation values of this reduced Hamiltonian are exactly the same as for the full model.
But, the problem is one can only exactly construct the Schmidt basis if one already knows the true ground state.
So instead one uses a mean field [Hartree-Fock] Hamiltonian and wave function for the bath. It looks like there is one free parameter, an effective chemical potential. That parameter is determined by a self-consistency condition that makes sure the one-electron density matrix calculated with the bath Hamiltonian is as close as possible to the impurity Hamiltonian. This is the analogue of the self-consistency condition of DMFT.

Some of the results look promising with favourable comparison with exact results for one dimensional Hubbard model obtained using the Bethe ansatz.

Given the low computational cost it is not clear why results were not obtained for larger clusters beyond 2 x 2.

I would be nice to see a longer paper, e.g. a PRB, with more details including a few worked examples, such as those I heard Garnett Chan mention briefly in a talk at Telluride.

All calculations presented are for zero temperature.
Cursory comments are made about it being straight-forward to extend to non-zero temperature. It is very important that this is done. One of the great achievements of DMFT is that it describes the temperature dependence of transport properties, including the crossover with increasing temperature from a Fermi liquid to a bad metal.

I am not sure about the results presented for spectral functions. It is claimed that they capture the "three peak structure" of DMFT but I fail to see that. In DMFT in the metallic phase near the Mott transition one sees a central peak [Kondo resonance] that is clearly separated from the upper and lower Hubbard bands. A sample is below, which also highlights the strong temperature dependence, taken from this preprint.

We dunno nothin' ...

We don't know anything about .... water, high-Tc superconductors, glasses, photosynthesis, enzymes, protein folding ....
We don't understand them. No one has any idea how they work. We have no theory. They are unsolved problems. No one can agree on anything....

Sometimes I hear strong claims such as this.
I think they are exaggerations. They diminish/ignore/dis-respect significant progress and understanding that has been made. 

Unfortunately, these claims of ignorance are often made by people who claim they are going to solve one of these problems, .... once you give them lots of money.....

Let me take one specific case: cuprate superconductors.
There are many things we know and understand that we did not when they were first discovered.

We have a phenomenological theory for all the "macroscopic" phenomena: Ginzburg-Landau!

Although not everyone agrees I think it is fair to say that the essential physics is in a one-band Hubbard model and the key physics is:
strong electronic correlations,
a doped antiferromagnetic Mott insulator,
d-wave pairing that is "mediated"/caused from some mixture/variant of "antiferromagnetic" spin fluctuations or RVB spin singlets,.....

We certainly don't understand the cuprates at the same level as elemental superconductors. But we do understand some things.

We certainly don't understand water at the same level as liquid argon. But we do understand some things.

This post was partly stimulating by reading Biman Bagchi's response to such a claim about water in his recent book.

There are still outstanding challenges and these topics should attract ongoing attention.

I don't think the topics I listed above are anything like problems such as dark energy, dark matter, quantum gravity, ... or superconductivity before BCS
and then there is consciousness....

Am I over-reacting?

Aside: the title of this post is from this music video. Some think it is hilarious. I find it a bit too close to the truth.