Angle-Dependent Magnetoresistance as a probe of Fermi surface properties in cuprates

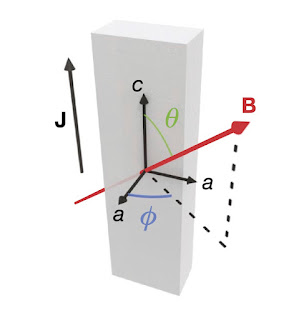
About twenty-five years ago I became interested in how the Fermi surface of the metallic state of organic charge-transfer salts could be mapped out by measuring the interlayer resistance as a function of the direction of a large applied magnetic field. [A nice review from 2004 is by Mark Kartsovnik]. Later this technique was used for a range of other metals including strontium ruthenate, iron pnictides, semiconductor heterostructures, and finally cuprates, mostly in the overdoped region.

For the cuprates, it was discovered that one could not only map out the shape of the intralayer Fermi surface, but also anisotropies in the scattering rate and the interlayer hopping integral. Of particular interest was the finding that the overdoped cuprates were not simple Fermi liquids, as usually claimed, but more like anisotropic marginal Fermi liquids.

It should be stressed that the Fermi surface information is extracted indirectly by comparing experimental curves of angle-dependence to calculations based on different models for the shape of the Fermi surface, anisotropies in the scattering rate, and interlayer hopping. Thus, there is a fair bit of curve fitting to determine the parameters of the model. However, when one has observations at several magnetic fields, temperatures, and curves for the angle dependence in all directions, there are a lot of constraints, and specific anisotropies tend to produce some specific qualitative features in the shapes of the curves. Examples are shown below, taken from the Nature paper referenced below.

Recently, measurements have been reported on samples of the cuprate Nd-LSCO 

[La1.6−xNd0.4SrxCuO4] at dopings of p=0.21 and p=0.24, lying on both sides of the putative quantum critical point at p=0.23. 

The differences between the ADMR at these two dopings are analysed quantitatively in a preprint, which claims to show that at p=0.21 the Fermi surface is reconstructed due to (pi,pi) ordering. This is important as it relates to the fundamental question as to the origin of the pseudogap state.

Fermi surface transformation at the pseudogap critical point of a cuprate superconductor

Yawen Fang, Gael Grissonnanche, Anaelle Legros, Simon Verret, Francis Laliberte, Clement Collignon, Amirreza Ataei, Maxime Dion, Jianshi Zhou, David Graf, M. J. Lawler, Paul Goddard, Louis Taillefer, B. J. Ramshaw

Submitted on 3 Apr 2020 (v1), last revised 26 Nov 2020 (v2)

Aside: There is also a Nature paper, Linear-in temperature resistivity from an isotropic Planckian scattering rate, by the same group that compares the p=0.24 observations to those on the overdoped cuprate Tl2201 [p=0..29]. The arxiv notes "substantial text overlap" between the preprint above and the preprint for the Nature paper. [Figure 2 in v1 of the preprint above is in the Nature paper].

Here I focus on the first preprint as it stimulated a nice theory preprint

Interpreting Angle Dependent Magnetoresistance in Layered Materials: Application to Cuprates

Seth Musser, Debanjan Chowdhury, Patrick A. Lee, T. Senthil

They present a strong case against the main claim of Fang et al. that their ADMR data supports a reconstructed Fermi surface for the p=0.21 system.

There are several nice things about this preprint.

1. It shows how one should be careful about interpreting ADMR

2. It highlights the possible role of an anisotropic quasi-particle weight, Z(phi), where phi denotes the position on the intralayer Fermi surface, not the direction of the field. Anisotropy can arise from correlation effects and or "coherence factors" associated with Fermi surface reconstruction due to an ordered state. 

2. In their modeling, Fang et al. did not include the effects of Z(phi) and Musser et al. show that when it is included the qualitative differences in the ADMR that they claim arise due to the ordered state do not appear.

3. The authors consider a "toy" model for which some analytical results can be obtained. 

4. This provides some physical insight into the origins of the different features in the data, such as the peak around theta=40 degrees [It is just the magic angle associated with the average radius of the Fermi surface] and how the behaviour near theta=90 degrees depends on the relative size of different parameters [see especially equation (16)].

5. What is happening in this material may not be generic to the cuprates. "The van Hove filling in Nd-LSCO is located between the two dopings, p = 0.21 and p = 0.24, respectively. Thus what was a large Fermi surface centered at the Γ-point on the overdoped side will become a Fermi surface centered at (π, π) on the underdoped side, assuming no reconstruction occurs"

6. The most important insight is at the beginning of Section V. When the value of of the interlayer hopping integral t_perp(phi) averaged over the Fermi surface, changes from non-zero to zero an upturn in the ADMR at low angles (i.e. fields almost parallel to the layers) to a downturn. This suggests an alternative explanation for the transition seen in the preprint.

7. It highlights the often overlooked fact that observation of ADMR is not conclusive evidence of a three-dimensional Fermi surface. Using the parameters from the experimental preprint gives typical values of t_perp * tau ~ 0.1, and so the materials are far from the regime of a coherent three-dimensional Fermi surface.

I have a few minor comments

a. Like many others, the authors incorrectly credit with Yamaji explaining the magic angles associated with ADMR. However, Yamaji's explanation is not the correct one because it involves quantised orbits, whereas the effect is semi-classical, as explained by Kartsovnik, Laukhin, Pesotskii, Schegolev, and Yakovenko. 

b. Investigation of the role of small closed orbits when the magnetic field is almost parallel to the layers is credited to Schofield and Cooper. However, there was earlier and more detailed work by Hanasaki et al. Albeit, both of these papers consider the clean high field limit and so are of debatable relevance.

c. It would be nice to know the status of Fang et al., preprint on which this paper is based, particularly as the first authors of both are in the same department.