Quantum capacitance is the charge compressibility

In a quantum many-body system a key thermodynamic quantity is the charge compressibility, the derivative of the charge density with respect to the chemical potential d n/d mu.

In a degenerate Fermi gas kappa is simply proportional to the density of states at the Fermi energy.
kappa is zero in a Mott insulator. As the Mott transition is approached, the behaviour of kappa is non-trivial. For a band width (or frustration) controlled Mott transition that occurs at half filling, Jure Kokalj and I showed how kappa smoothly approached zero as the Mott transition was approached. However, there is some debate as to what happens for doping controlled transitions, e.g. does kappa diverge as one approaches the Mott insulator.

One thing I had wondered about was how one actually measures kappa accurately in an experiment. Varying the chemical potential and/or charge density is not always possible or straightforward.
See for example, Figure 10 in this review by Jaklic and Prelovsek, which compares experimental results on the cuprates with calculations for the t-J model.
The experimental error bars are large.

At the recent Australasian Workshop on Emergent Quantum Matter, Chris Lobb gave a nice talk about "Atomtronics" where he talked about how you define R, L, and C [resistance, inductance, and capacitance] in cold atom transport experiments. He argued that the capacitance was related to
d mu/dn. This got my attention.
Michael Fuhrer pointed out how there were measurements of the "quantum capacitance" for graphene.

Recent measurements for graphene are in this nice paper. They give a helpful review of the technique and the relevant equations.

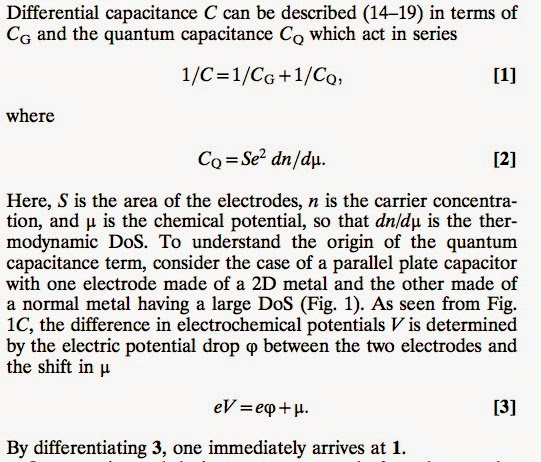
Personally, I don't find this "derivation" completely obvious, but can follow the algebraic steps.

Why is C_Q called the "quantum capacitance"? This wasn't at all clear to me.
But, there is a helpful discussion in a paper,
Quantum capacitance devices by Serge Luryi

For a non-interacting two dimensional fermion gas the density of states scales inversely with the square of hbar. Hence, it diverges as hbar goes to zero. This means the quantum capacitance diverges and the "quantum" term C_Q in the equation [1] disappears.

In the graphene paper, the authors extract the renormalised Fermi velocity as a function of the density [band filling] and compare to renormalisation group calculations, that take into account electron-electron interactions, and predict a logarithmic dependence, as shown below. Red and blue are the theory, and experimental curves, respectively.

As exciting as the above is, it is still not clear to me how to measure the "quantum capacitance" for a multi-layer system.

Update. (December 10, 2014)
Lu Li brought to my attention his measurements of the capacitance of the 2DEG at the LaAlO₃/SrTiO₃ interface, and earlier measurements of negative compressibility of correlated two dimensional electron gases (2DEGs) by Eisenstein and by Kravchenko.
Background theory has been discussed extensively by Kopp and Mannhart.